Solvation

Solvation free energy is an important quantity in Computational Chemistry with a variety of applications, especially in drug discovery and design, and for protein docking. Since all biochemical processes require the presence of water, solvation effects play a major role that therefore has to be modelled correctly in order to achieve reliable and meaningful predictions.

Unfortunately, the very properties making water the perfect solvent, namely its polar nature, high dielectric constant, and its ability to form hydrogen bonds, are major obstacles in the accurate prediction of solvation effects. The accurate prediction of solvation free energies of biomolecules in water is therefore still a largely unsolved problem.

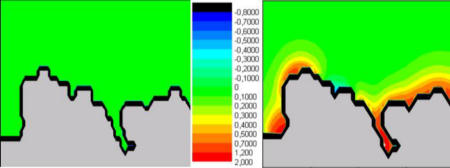
The most successful models for predicting the polar contribution to solvation free energies of small molecules (and proteins) employ the Poisson or Poisson-Boltzmann equations. Within this approach, the solvent is typically treated as a structureless medium of a given dielectric constant (for water, ε ≈ 80). The solute itself is treated as a low-ε cavity.

Clearly, this approach fails to take into account many relevant details of the solvent structure, and therefore induces errors in the quantitative determination of solvation effects. The presence of long-ranged electrostatic fields induces static correlations among the polar molecules that may vary considerably in space depending on the molecular build-up of the solute. These can be taken into account by perfoming Molecular Dynamics simulations, thus by leaving the continuum description at the expense of high computational cost even for small molecules.

Including more structural detail into the continuum description on the one hand without increasing computational complexity on the other is therefore highly desirable. At the same time, however, also the avoidance of additional parameter sets, which is common practice in the use of continuum electrostatics in computational chemistry, is a key requirement.

In our approach, we follow one particular route to achieve this goal. We develop an approach for the computation of the electrostatic (polar) contribution to the free energy of solvation based on nonlocal electrostatics. This continuum approach, originating in the physics literature as a phenomenological generalization of classical electrostatics, is developed within a physically transparent minimal parameter set.

We have tested our approach on simple ions and small molecules for which both experimental results and other theoretical descriptions are available for quantitative comparison, and find that our approach is both physically transparent and quantitatively reliable. The results have just been submitted for publication. Currently, we extend and apply our approach to protein docking and to the gating behaviour of ion channels. We are also at the moment investigating the implications of the more realistic potentials in nonlocal theory for the process of molecular recognition.